Debye's Contribution to Specific Heat Theory Einstein's oscillator treatment of specific heat gave qualitative agreement with experiment and gave the correct high temperature limit (the Law of Dulong and Petit). The quantitative fit to experiment was improved by Debye's recognition that there was a maximum number of modes of vibration in a solid. He pictured the vibrations as standing wave modes in the crystal, similar to the electromagnetic modes in a cavity which successfully explained blackbody radiation. The density of states for these modes, which are called "phonons", is of the same form as the photon density of states in a cavity. To impose a finite limit on the number of modes in the solid, Debye used a maximum allowed phonon frequency now called the Debye frequency υD. 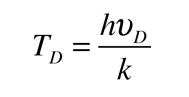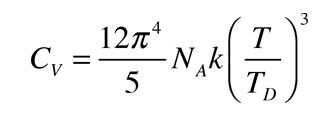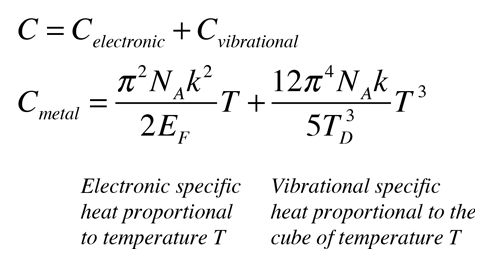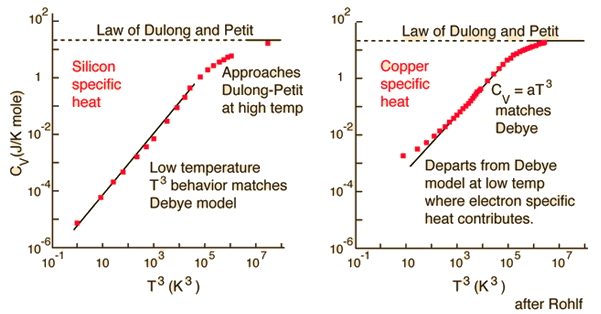In the treatment of specific heat, we define a Debye temperature by For low temperatures, Debye's treatment led to a specific heat Show The dependence upon the cube of the temperature agreed with experimental results for nonmetals, and for metals when the electron specific heat was taken into account. The measurement of the low temperature specific heat variation with temperature has led to tabulation of the Debye temperatures for a number of solid materials. The full expression for the Debye specific heat must be evaluated by numerical procedures. It has the correct limiting values at both high and low temperatures. Define constants Table of specific heats Einstein-Debye specific heat expression Table of Debye temperatures

Index Reference Rohlf Ch 14. Blatt Sec. 4.3

HyperPhysics***** Thermodynamics R Nave

Go Back

Einstein-Debye Specific Heats The Einstein-Debye phonon model produced agreement with the low-temperature cubic dependence of specific heat upon temperature. Explaining the drastic departure from the Law of Dulong and Petit was a major contribution of the Einstein and Debye models. The final step in explaining the low temperature specific heats of metals was the inclusion of the electron contribution to specific heat. When these were combined, they produced the expression Note that the vibrational part is only the low temperature limit of the more general Debye specific heat. The data below show that the Debye phonon model with its cubic dependence on temperature matches the silicon data to very low temperatures. The copper shows a departure from the cubic dependence, showing evidence of electron specific heat. The vibrational term here is only the low temperature limit of the Debye specific heat expression; the full expression includes an integral which must be evaluated numerically. It produces good agreement with the transition to the Dulong and Petit limit at high temperatures. Note that the model for specific heat presented here uses both forms of quantum statistics. Bose-Einstein statistics is used to describe the contribution from lattice vibrations ( phonons), and Fermi-Dirac statistics must be used to describe the electron contribution to the specific heat.

Index References Blatt Sec 4.3. Rohlf Ch 14.

HyperPhysics***** Thermodynamics R Nave

Go Back