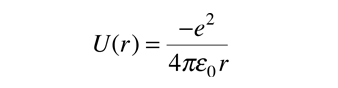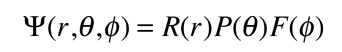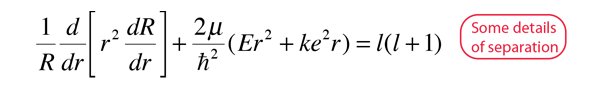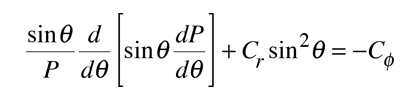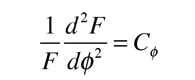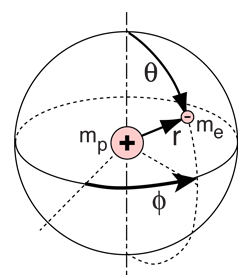
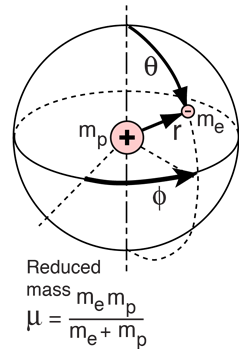
The solution of the Schrodinger equation for the hydrogen atom is a formidable mathematical problem, but is of such fundamental importance that it will be treated in outline here. The solution is managed by separating the variables so that the wavefunction is represented by the product: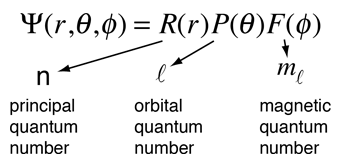

The separation leads to three equations for the three spatial variables, and their solutions give rise to three quantum numbers associated with the hydrogen energy levels.

The electron in the hydrogen atom sees a spherically symmetric potential, so it is logical to use spherical polar coordinates to develop the Schrodinger equation. The potential energy is simply that of a point charge:

The expanded form of the Schrodinger equation is shown below. Solving it involves separating the variables into the form

Similarly, a constant arises in the colatitude equation which gives the orbital quantum number: