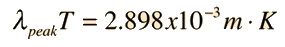Star Temperatures
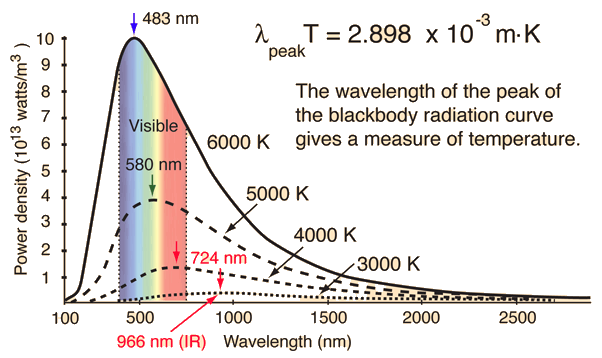
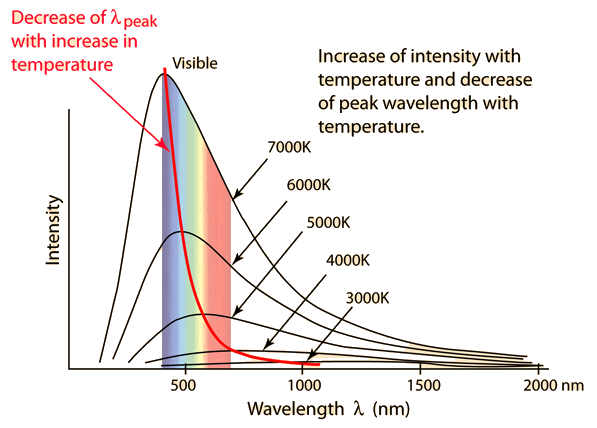
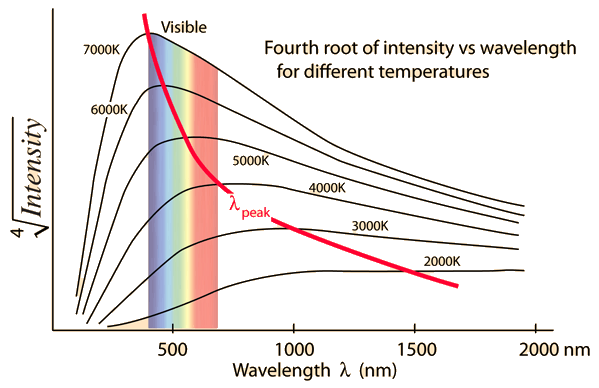
Stars approximate blackbody radiators and their visible color depends upon the temperature of the radiator. The curves show blue, white, and red stars. The white star is adjusted to 5270K so that the peak of its blackbody curve is at the peak wavelength of the sun, 550 nm. From the wavelength at the peak, the temperature can be deduced from the Wien displacement law.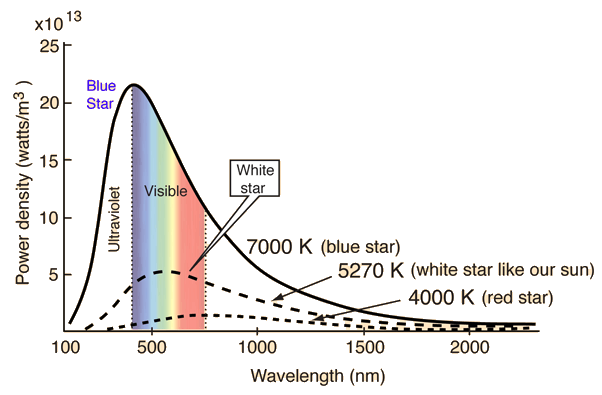
The change in the visual color of stars of different temperatures can be traced out on a chromaticity diagram.
Star classification
Blackbody radiation concepts
| HyperPhysics***** Quantum Physics | R Nave |