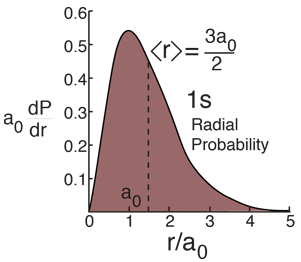
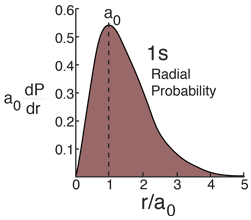
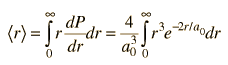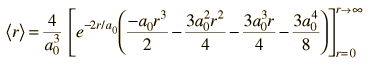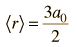The Most Probable Radius
Hydrogen Ground State

The radial probability density for the hydrogen ground state is obtained by multiplying the square of the wavefunction by a spherical shell volume element. 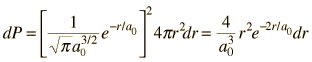It takes this comparatively simple form because the 1s state is spherically symmetric and no angular terms appear.

Dropping off the constant terms and taking the derivative with respect to r and setting it equal to zero gives the radius for maximum probability.

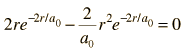
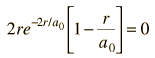
which gives

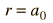
where

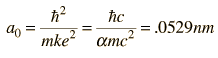
The most probable radius is the ground state radius obtained from the Bohr theory. The Schrodinger equation confirms the first Bohr radius as the most probable radius but goes further to describe in detail the profile of probability for the electron radius.

The Expectation Value for Radius
Hydrogen Ground State

The average or "expectation value" of the radius for the electron in the ground state of hydrogen is obtained from the integral

This requires integration by parts. The solution is

All the terms containing r are zero, leaving

It may seem a bit surprising that the average value of r is 1.5 x the first Bohr radius, which is the most probable value. The extended tail of the probability density accounts for the average being greater than the most probable value.