 |
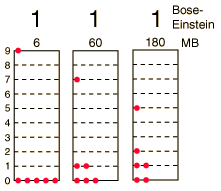
How many ways can you distribute 9 units of energy among 6 identical, indistinguishable bosons?The three distributions of particles at left each have the same energy, the same kind of particles, and the same number of particles. If the particles were distinguishable particles and described by Maxwell-Boltzmann statistics, then the distribution at right would be 30 times more likely than the one on the left because there are 30x as many distinguishable ways to produce it. But if they are indistinguishable bosons, the three states are equally probable and given a statistical weight of 1. This means that instead of 2002 distinguishable microstates arising from 26 macrostates, there are only 26 states. |
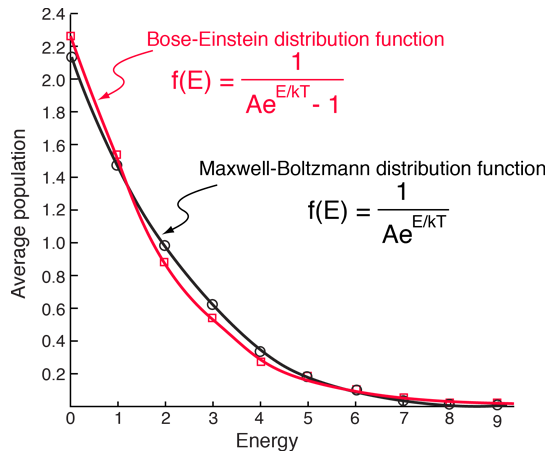
Evaluating the average occupancy of each energy state is much simpler than in the Maxwell-Boltzmann example since each macrostate has a weight of 1. The average occupancy is just the sum of the numbers of particles in a given energy state over all the 26 distributions divided by 26.
| Evaluate the average for each energy state. | Compare with Maxwell-Boltzmann example. |
Reference
Blatt
Ch. 11
| HyperPhysics***** Quantum Physics | R Nave |