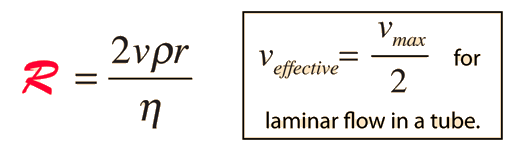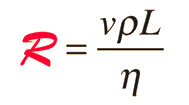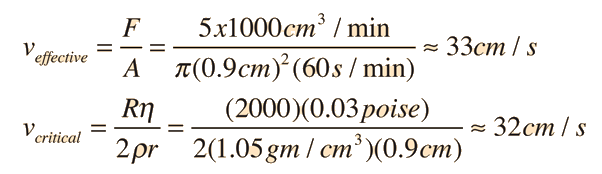Turbulent Flow

Flow descriptions such as Poiseuille's law are valid only for conditions of laminar flow. At some critical velocity, the flow will become turbulent with the formation of eddies and chaotic motion which do not contribute to the volume flowrate. This turbulence increases the resistance dramatically so that large increases in pressure will be required to further increase the volume flowrate. Experimental studies have characterized the critical velocity for a long straight tube in the form

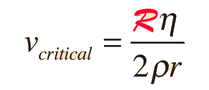
which depends upon the viscosity η in poise, the density ρ in gm/cm³ , the radius of the tube r in cm. The script R is an experimental constant called the Reynold's number. The reported Reynolds number for blood flow is about 2000. Modeling blood flow in the human aorta according to this criterion leads to the expectation of some turbulence in the center of the flow.

Reynolds Number

The parameters are viscosity η, density ρ and radius r. The suggested number of 2000 is used for the application to blood flow for the example of the aorta. Another approach is to define a variable Reynolds number in terms of the maximum velocity for laminar flow in a tube by

and characterize the condition for turbulence as the condition when the Reynolds number reaches a critical value like 2000. For a more general approach to turbulence when objects move through a fluid, the relation takes the following form:

where L is a characteristic length associated with the object.

Turbulence in flow is not something that can be calculated precisely, but the concept of the Reynolds number is a helpful one for general engineering of fluid flow problems. It can have some predictive power when measurements are made for a particular geometry to establish an experimental Reynolds number, and can then be helpful in scaling up the size of that geometry.

References:

Encyclopedia of Science, Darling

Reynolds Number, NASA

Turbulent Flow in Aorta?

Blood flow in the human body is remarkably free of turbulence , but sounds attributed to turbulence are sometimes detected by stethescope associated with the aorta. Modeling the flow makes use of the Reynold's number and the associated critical velocity. Assuming a nominal blood volume flowrate of 5 liters/min and a radius of 0.9 cm for the aorta:

But when the velocity profile for tube flow is taken into account, it is found that the maximum velocity of flow is twice the effective value, so a velocity of 66 cm/s would be expected to produce turbulence in the center of the aorta.

Non-Newtonian Fluids