Buoyancy
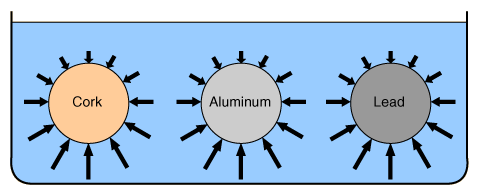
Buoyancy arises from the fact that fluid pressure increases with depth and from the fact that the increased pressure is exerted in all directions (Pascal's principle) so that there is an unbalanced upward force on the bottom of a submerged object.
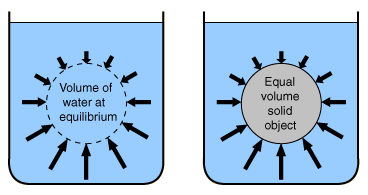 |
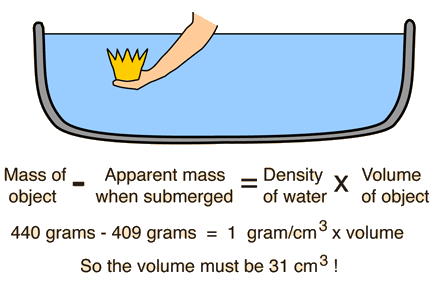
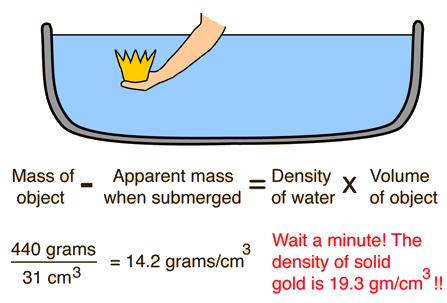
Since the "water ball" at left is exactly supported by the difference in pressure and the solid object at right experiences exactly the same pressure environment, it follows that the buoyant force on the solid object is equal to the weight of the water displaced (Archimedes' principle). |
Objects of equal volume experience equal buoyant forces.
Applications of buoyancy.
Buoyancy concepts
| HyperPhysics***** Mechanics ***** Fluids | R Nave |