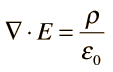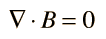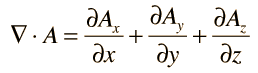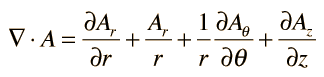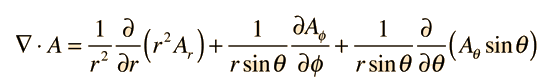The Divergence

The divergence of a vector field

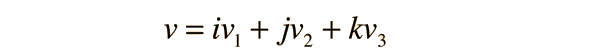
in rectangular coordinates is defined as the scalar product of the del operator and the function

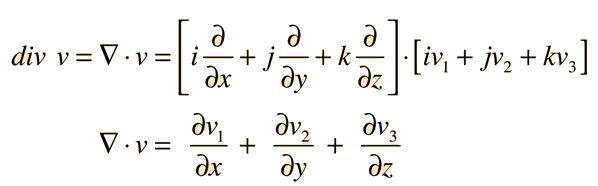
The divergence is a scalar function of a vector field. The divergence theorem is an important mathematical tool in electricity and magnetism.

R Nave

Applications of Divergence

The divergence of a vector field is proportional to the density of point sources of the field. In Gauss' law for the electric field

the divergence gives the density of point charges. In Gauss' law for the magnetic field

the zero value for the divergence implies that there are no point sources of magnetic field.

R Nave

Compared to the divergence in rectangular coordinates:

In cylindrical polar coordinates:

and in spherical polar coordinates:

R Nave