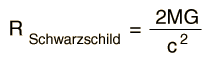Black Hole Conditions

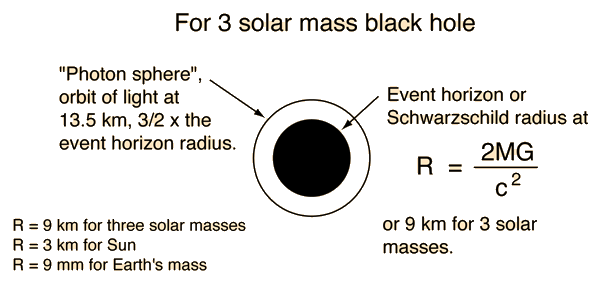
After collapse to the neutron star stage, stars with masses less than 2-3 solar masses should remain neutron stars, gradually radiating away their energy, because there is no known mechanism for further combination, and forces between neutrons prevent further collapse. But this neutron force is the last stand, and our best calculations indicate that this repulsion which prevents collapse cannot withstand the gravity force of masses greater than 2 to 3 solar masses. Such neutron stars would collapse toward zero spatial extent - toward a "singularity". Once they collapsed past a certain radius, the "event horizon", then even light could not escape: black hole. Since black holes by their very definition cannot be directly observed, proving their existence is difficult. The indirect evidence for the black hole Cygnus X-1 is a good example of the search for black holes.

R Nave

Go Back

The Schwarzschild Radius

Any mass can become a black hole if it collapses down to the Schwarzschild radius - but if a mass is over some critical value between 2 and 3 solar masses and has no fusion process to keep it from collapsing, then gravitational forces alone make the collapse to a black hole inevitable. Down past electron degeneracy, on past neutron degeneracy and then on past the Schwarzchild radius to collapse toward zero spatial extent - the singularity. The Schwarzschild radius (event horizon) just marks the radius of a sphere past which we can get no particles, no light, no information.

Gravity and the photon

Black hole conditions

Black hole description

Index

Black hole concepts

HyperPhysics***** Astrophysics

R Nave

Go Back

Black Hole Description

One of the terms used with black holes is "photon sphere", the radius of the orbit of light around the black hole. For 3 solar masses this radius is 13.5 km, 3/2 x the event horizon radius. The event horizon radius is also called the Schwarzschild radius.

Calculation

Index

Black hole concepts

HyperPhysics***** Astrophysics

R Nave

Go Back