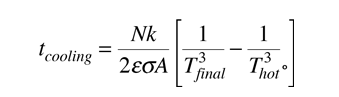Radiative Cooling Time
The rate of radiative energy emission from a hot surface is given by the Stefan-Boltzmann law .
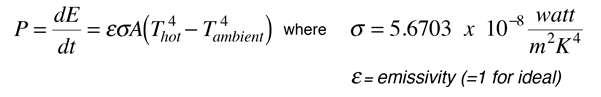
Here P is the power emitted from the area, and E is the energy contained by the object. For very hot objects, the role of the ambient temperature can be neglected. If the hot temperature is more than 3.16 times the ambient, then the contribution of ambient terms is less than 1%. For example, for 300K ambient on the earth, an object of temperature higher than 1000K can be treated like a pure radiator into space. If the heat loss is purely radiative and not limited by heat transfer to the radiating surface, then the cooling time can be modeled for a hot object.
If the energy of the object can be characterized by pure translational kinetic energy according to equipartition of energy, then
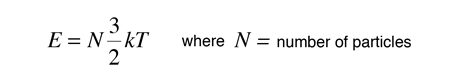
Using the chain rule for differentiation
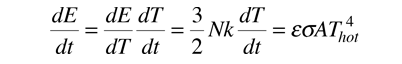
Rearranging gives us
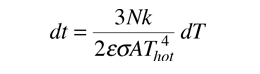
and integrating gives the cooling time
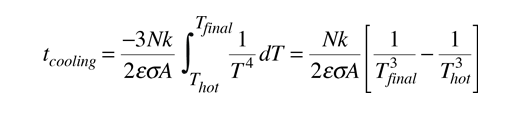
It must be kept in mind that for macroscopic objects, the calculated cooling time for the object as a whole will always be shorter than the real cooling time, so that it gives a lower bound. The above relationship assumes infinite thermal conductivity so that the temperature of the whole object is equal to the surface temperature. In the real world, the surface will cool faster than the interior. The rate of heat transfer from the interior will be expected to limit the rate of radiative loss from the surface.
| Modeling the Radiative Cooling of a Hot Sphere |
| Modeling the radiative cooling of a volcanic fragment |
| Kelvin Cooling Time for the Earth |
| HyperPhysics***** Thermodynamics | R Nave |