Reflection of Sound

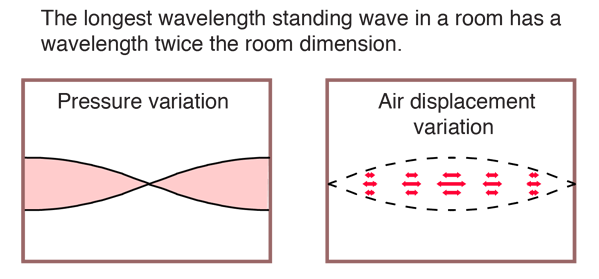
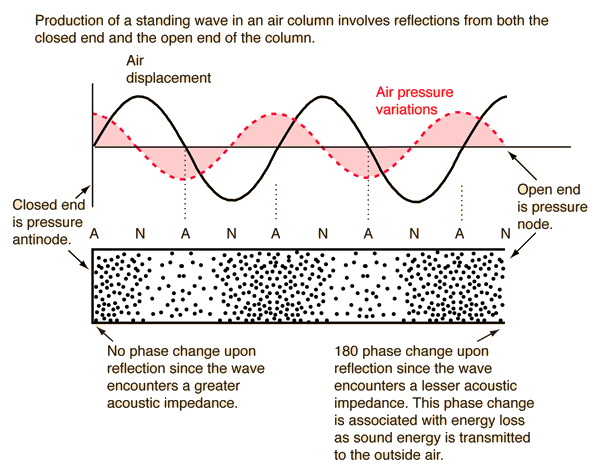
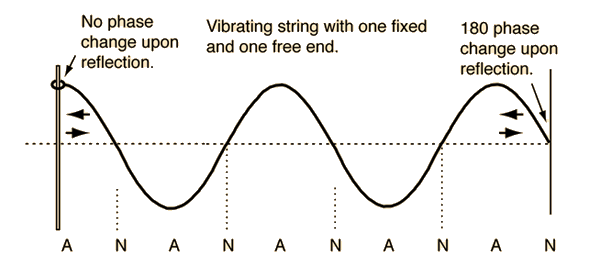
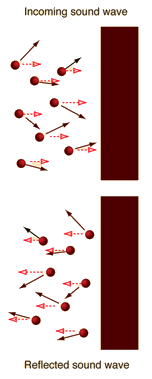
The reflection of sound follows the law "angle of incidence equals angle of reflection", sometimes called the law of reflection. The same behavior is observed with light and other waves, and by the bounce of a billiard ball off the bank of a table. The reflected waves can interfere with incident waves, producing patterns of constructive and destructive interference. This can lead to resonances called standing waves in rooms. It also means that the sound intensity near a hard surface is enhanced because the reflected wave adds to the incident wave, giving a pressure amplitude that is twice as great in a thin "pressure zone" near the surface. This is used in pressure zone microphones to increase sensitivity. The doubling of pressure gives a 6 decibel increase in the signal picked up by the microphone. Reflection of waves in strings and air columns are essential to the production of resonant standing waves in those systems.

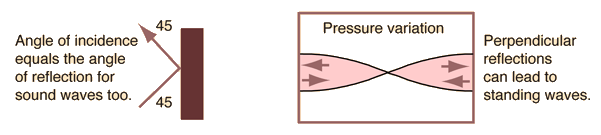
Examples of reflection

Plane wave, plane surface

Point source, plane surface

Point source, concave surface

Phase Change Upon Reflection

The phase of the reflected sound waves from hard surfaces and the reflection of string waves from their ends determines whether the interference of the reflected and incident waves will be constructive or destructive. For string waves at the ends of strings there is a reversal of phase and it plays an important role in producing resonance in strings. Since the reflected wave and the incident wave add to each other while moving in opposite directions, the appearance of propagation is lost and the resulting vibration is called a standing wave.

When sound waves in air (pressure waves) encounter a hard surface, there is no phase change upon reflection. That is, when the high pressure part of a sound wave hits the wall, it will be reflected as a high pressure, not a reversed phase which would be a low pressure. Keep in mind that when we talk about the pressure associated with a sound wave, a positive or "high" pressure is one that is above the ambient atmospheric pressure and a negative or "low" pressure is just one that is below atmospheric pressure. A wall is described as having a higher "acoustic impedance" than the air, and when a wave encounters a medium of higher acoustic impedance there is no phase change upon reflection.

On the other hand, if a sound wave in a solid strikes an air boundary, the pressure wave which reflects back into the solid from the air boundary will experience a phase reversal - a high-pressure part reflecting as a low-pressure region. That is, reflections off a lower impedance medium will be reversed in phase.

Besides manifesting itself in the "pressure zone" in air near a hard surface, the nature of the reflections contribute to standing waves in rooms and in the air columns which make up musical instruments.

The conditions which lead to a phase change on one end but not the other can also be envisioned with a string if one presumes that the loose end of a string is constrained to move only transverse to the string. The loose end would represent an interface with a smaller effective impedance and would produce no phase change for the transverse wave. In many ways, the string and the air column are just the inverse of each other.

Pressure Zone

The sound intensity near a hard surface is enhanced because the reflected wave adds to the incident wave, giving a pressure amplitude that is twice as great in a thin "pressure zone" near the surface. This is used in pressure zone microphones to increase sensitivity. The doubling of pressure gives a 6 decibel increase in the signal picked up by the microphone.

This is an attempt to visualize the phenomenon of the pressure zone in terms of the dynamics of the air molecules involved in transporting the sound energy. The air molecules are of course in ceaseless motion just from thermal energy and have energy as a result of the atmospheric pressure. The energy involved in sound transport is generally very tiny compared to that overall energy. If you visualize the velocity vectors shown in the illustration as just that additional energy which associated with the sound energy in the longitudinal wave, then we can argue that the horizontal components of the velocities will just be reversed upon collision with the wall. Presuming the collisions with the wall to be elastic, no energy is lost in the collisions. Viewing the collection of molecules as a "fluid", we can invoke the idea that the internal pressure of a fluid is a measure of energy density. The energy of the molecules reflecting off the wall adds to that of the molecules approaching the wall in the volume very close to the wall, effectively doubling the energy density and hence the pressure associated with the sound wave.