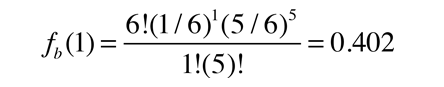Statistics of Dice Throw
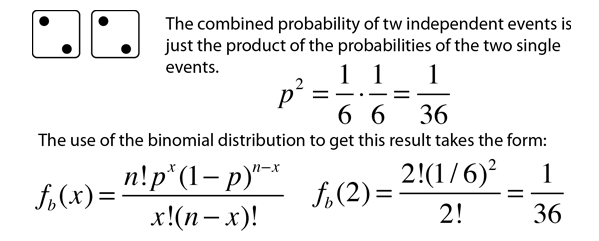
The probababilities of different numbers obtained by the throw of two dice offer a good introduction to the ideas of probability. For the throw of a single die, all outcomes are equally probable. But in the throw of two dice, the different possibilities for the total of the two dice are not equally probable because there are more ways to get some numbers than others. There are six ways to get a total of 7, but only one way to get 2, so the "odds" of getting a 7 are six times those for getting "snake eyes". This simple example raises the idea of distinguishable states. For example, throwing a 3 is twice as likely as throwing a 2 because there are two distinguishable ways to get a 3.
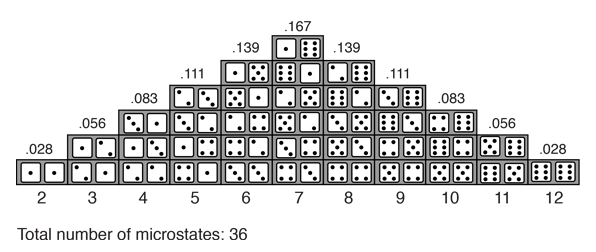
The probability of getting a given value for the total on the dice may be calculated by taking the total number of ways that value can be produced and dividing it by the total number of distinguishable outcomes. So the probability of a 7 on the dice is 1/6 because it can be produced in 6 ways out of a total of 36 possible outcomes.
| Dice and the Binomial Distribution |
Statistics concepts
| HyperPhysics*****HyperMath | R Nave |