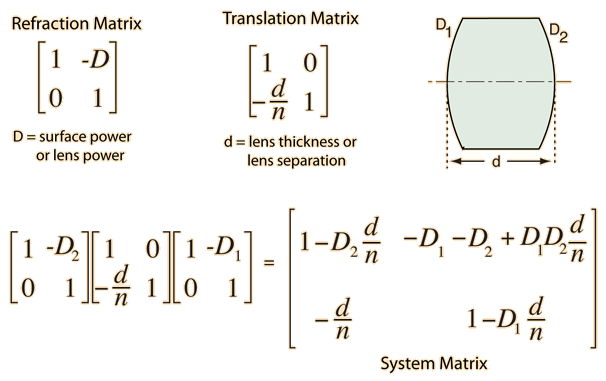
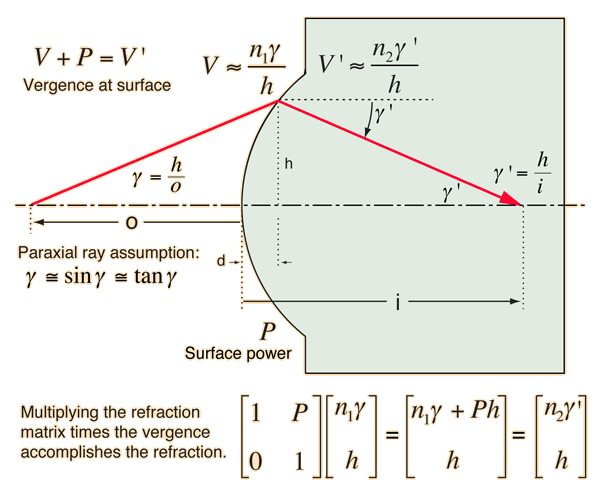
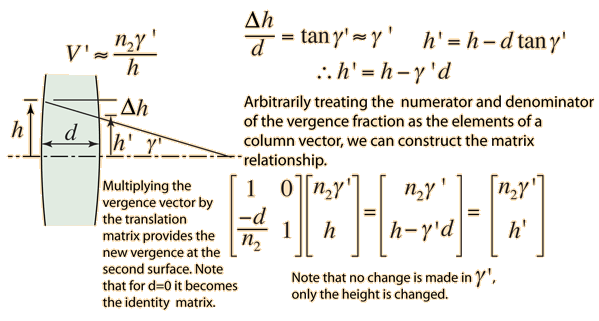
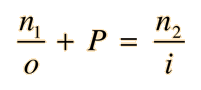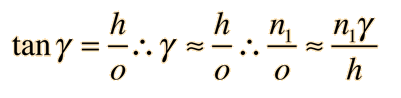System Matrix, Hecht Form

For systems of multiple thick lenses, it is sometimes useful to represent the system by a system matrix. The matrix is built up by multiplying the refraction matrices and translation matrices.

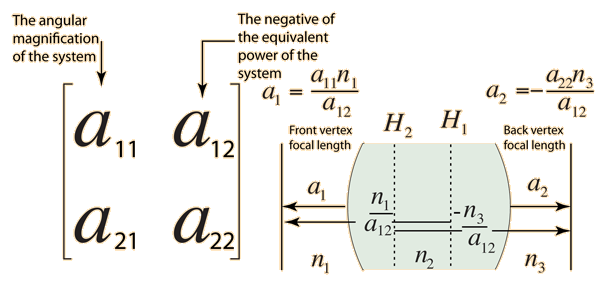
This is the form of the system matrix used by Hecht. An alternate form is used by Meyer-Arendt.

Refraction and Translation Matrices

Hecht form

Refraction Matrix

The use of vergence to describe the refraction at an interface can be used as a starting point for developing a matrix to perform the refraction.

The vergence relationship is

and for small angles (paraxial approximation):

Arbitrarily treating the numerator and denominator of the vergence fraction as the elements of a column vector, we can construct the matrix relationship for refraction.

Translation Matrix

The geometry of translation across the thickness of a lens can be put in matrix form for rays satisfying the paraxial approximation.