Principle of Equivalence

While attributing a kind of "effective mass" to the photon is one way to describe why the path of light is bent by a gravity field, Einstein's approach in general relativity is to associate a mass with a curvature of space-time, i.e. the existence of a mass will produce a curvature in space-time around it. From the point of view that light will follow the shortest path, or follows a geodesic of space-time, then if the Sun curves the space around it then light passing the Sun will follow that curvature.

Advance of Mercury's Perihelion

The perihelion of the orbit of the planet Mercury advances 2 degrees per century. 80 seconds of that advance was accounted for by perturbations from the other planets, etc., but the last 40 seconds of arc were unaccounted for. General relativity predicts an additional 43 seconds of arc and was one of the first triumphs of Einstein's theory. The eccentricity of Mercury's orbit is exaggerated here to emphasize the effect. More numerical detail

Advance of Mercury's Perihelion

According to Roy D. North, more precise numbers are as follows (in arcsec per Julian century): 5599 total advance with respect to to geocenter (our reference frame.) 5025 contribution of precession of Earth's equinoxes. 531 Classical or Newtonian contribution of the other planets. 43 General relativity correction (modern theory: 42.98)

Gravitational Deflection of Light

Einstein's calculations in his newly developed general relativity indicated that the light from a star which just grazed the sun should be deflected by 1.75 seconds of arc. It was tested during the total eclipse of 1919 and during most of those which have occurred since.

This bending of light can produce a gravitational lensing effect if a distant galaxy or quasar is closely aligned with a massive galaxy closer to us. If one galaxy is directly behind another, the result can be a circle of light called an Einstein ring.

Light Deflection and Space-time Curvature

This is a common approach to try to gain some visualization of the curvature of space-time by a gravitational mass. It starts by depicting space as a two-dimensional elastic sheet. If a massive ball is placed on this sheet, it will produce an indentation or curvature. If a smaller ball is rolled by the larger one, its path will be deflected by the indentation of the larger ball. While not adequate to depict the curvature of 4-dimensional space-time, it at least is a start. The bending of light above is greatly exaggerated.

Einstein's calculations in his newly developed general relativity indicated that the light from a star which just grazed the sun should be deflected by 1.75 seconds of arc. It was measured by Eddington during the total eclipse of 1919 and has been reaffirmed during most of those which have occurred since.

This bending of light can produce a gravitational lensing effect if a distant galaxy or quasar is closely aligned with a massive galaxy closer to us. If one galaxy is directly behind another, the result can be a circle of light called an Einstein ring.

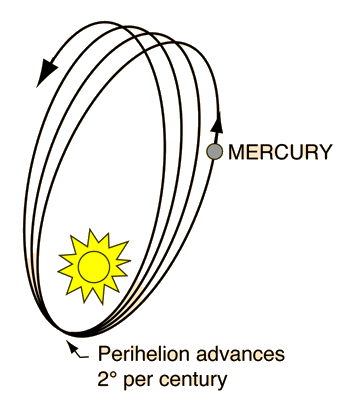
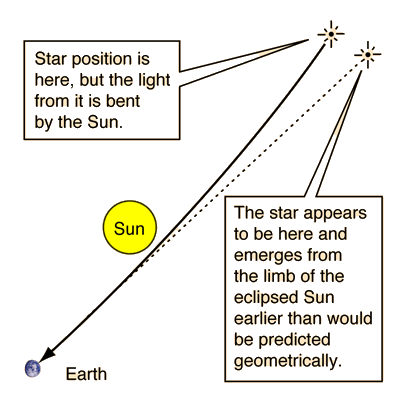
Eddington's Measurement of Light Deflection

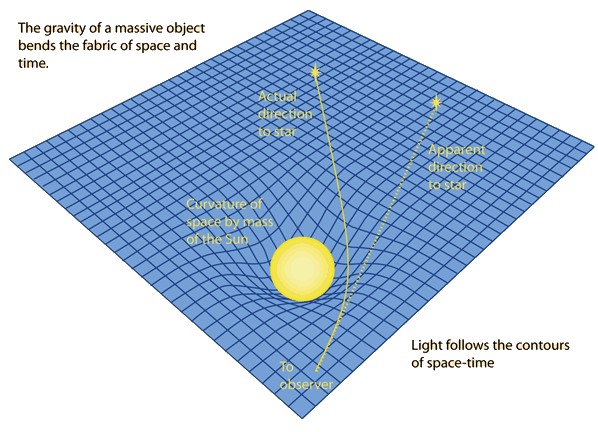
Einstein's calculations in his newly developed general relativity indicated that the light from a star which just grazed the sun should be deflected by 1.75 seconds of arc. The depiction of this bending is greatly exaggerated in the illustration above.

Einstein, writing in a German journal during World War I, suggested that during a total solar eclipse the deflection should be observable in terms of the time of emergence of a star from behind the Sun. Fraknoi, et al. comment that a copy of that paper came through neutral Holland and reached British astronomer Arthur S. Eddington. An expedition was organized to attempt to measure this deflection during the next total solar eclipse on May 29, 1919 at two locations: the island of Principe off the west coast of Africa and Sobral, Brazil. Photographs were obtained in both locations and confirmed the predicted deflection to about 20% accuracy.

Measurements during total solar eclipses have been made several times, notably by the Yerkes Observatory in 1953 and by the University of Texas in 1973. More accurate radio frequency measurements after that time became the standard approach to gravitational deflection of electromagnetic waves.