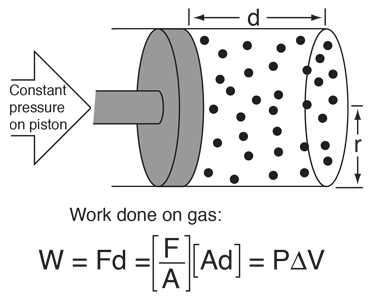
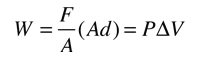Heat and Work Example
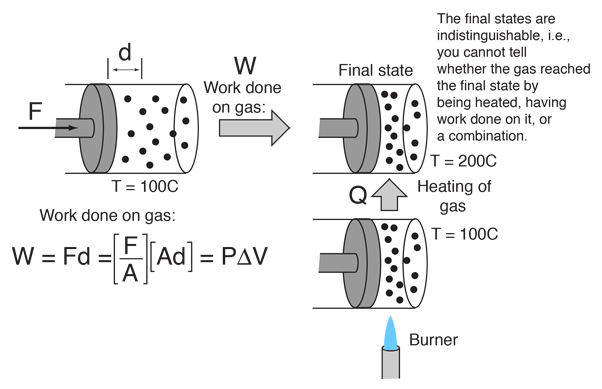
This example of the interchangeability of heat and work as agents for adding energy to a system can help to dispel some misconceptions about heat. I found the idea in a little article by Mark Zemansky entitled "The Use and Misuse of the Word 'Heat' in Physics Teaching". One key idea from this example is that if you are presented with a high temperature gas, you cannot tell whether it reached that high temperature by being heated, or by having work done on it, or a combination of the two.
To describe the energy that a high temperature object has, it is not a correct use of the word heat to say that the object "possesses heat" - it is better to say that it possesses internal energy as a result of its molecular motion. The word heat is better reserved to describe the process of transfer of energy from a high temperature object to a lower temperature one. Surely you can take an object at low internal energy and raise it to higher internal energy by heating it. But you can also increase its internal energy by doing work on it, and since the internal energy of a high temperature object resides in random motion of the molecules, you can't tell which mechanism was used to give it that energy.
In warning teachers and students alike about the pitfalls of misusing the word "heat", Mark Zemansky advises reflecting on the jingle:
|
"Teaching thermal physics
Is as easy as a song:
You think you make it simpler
When you make it slightly wrong."
| Zemansky's plea
Don't refer to the "heat in a body", or say "this object has twice as much heat as that body". He also objects to the use of the vague term "thermal energy" and to the use of the word "heat" as a verb, because they feed the misconceptions, but it is hard to avoid those terms. He would counsel the introduction and use of the concept of internal energy as quickly as possible.
|
Zemansky points to the First Law of Thermodynamics as a clarifying relationship. The First Law identifies both heat and work as methods of energy transfer which can bring about a change in the internal energy of a system. After that, neither the words work or heat have any usefulness in describing the final state of the sytem - we can speak only of the internal energy of the system.
|
Index
Internal energy concepts
Reference
Zemansky |