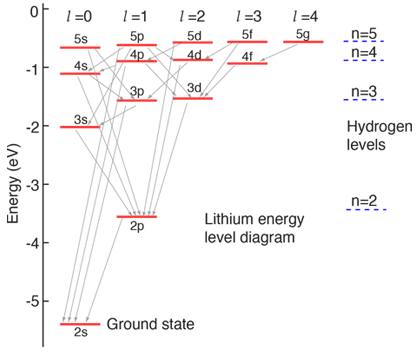
Lithium Energy Levels

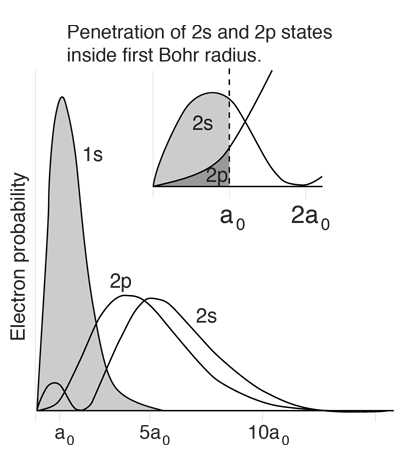
The lithium atom has a closed n=1 shell with two electrons and then one electron outside. Since the outer electron looks inward at just one net positive charge, it could be expected to have energy levels close to those of hydrogen. This is true for high angular momentum states as shown, but the s and p states fall well below the corresponding hydrogen energy levels.

The general layout here was taken from Rohlf but some discrepancies were found in the lower levels. The levels up through the 3d plus the 4p and 4d were rescaled to fit the data in the table of neutral lithium levels from NIST. Discrepancies may remain with the other levels. The ionization potential given by NIST was 5.395 eV.

Lithium Energy Levels:Orbital Dependence

The lithium 2s level is significantly lower than the 2p because of greater penetration past the shielding of the 1s electron. Both levels penetrate enough to be significantly lower than the n=2 hydrogen energy which they would have if the shielding were perfect. The 2s electron is lowered about 1.7 eV below the n=2 hydrogenic energy level of -3.4 eV which it would have if the shielding were perfect.