Quantum Harmonic Oscillator: Energy Minimum from Uncertainty Principle

The ground state energy for the quantum harmonic oscillator can be shown to be the minimum energy allowed by the uncertainty principle.

The energy of the quantum harmonic oscillator must be at least

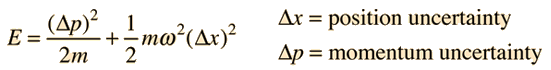
Taking the lower limit from the uncertainty principle

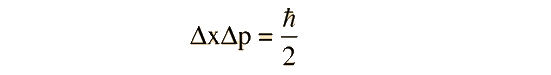
Then the energy expressed in terms of the position uncertainty can be written

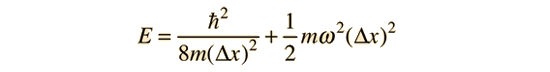
Minimizing this energy by taking the derivative with respect to the position uncertainty and setting it equal to zero gives

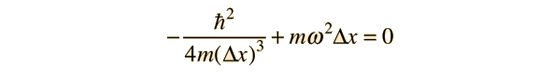
Solving for the position uncertainty gives

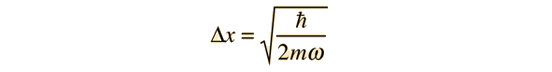
Substituting gives the minimum value of energy allowed.

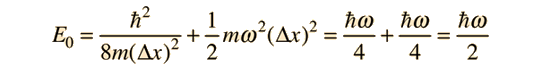
This is a very significant physical result because it tells us that the energy of a system described by a harmonic oscillator potential cannot have zero energy. Physical systems such as atoms in a solid lattice or in polyatomic molecules in a gas cannot have zero energy even at absolute zero temperature. The energy of the ground vibrational state is often referred to as "zero point vibration". The zero point energy is sufficient to prevent liquid helium-4 from freezing at atmospheric pressure, no matter how low the temperature.