Transition Probabilities and Fermi's Golden Rule

One of the prominent failures of the Bohr model for atomic spectra was that it couldn't predict that one spectral line would be brighter than another. From the quantum theory came an explanation in terms of wavefunctions, and for situations where the transition probability is constant in time, it is usually expressed in a relationship called Fermi's golden rule.

In general conceptual terms, a transition rate depends upon the strength of the coupling between the initial and final state of a system and upon the number of ways the transition can happen (i.e., the density of the final states). In many physical situations the transition probability is of the form

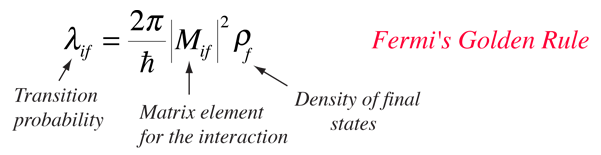
The transition probability λ is also called the decay probability or decay constant and is related to the mean lifetime τ of the state by λ = 1/τ. The general form of Fermi's golden rule can apply to atomic transitions, nuclear decay, scattering ... a large variety of physical transitions.

A transition will proceed more rapidly if the coupling between the initial and final states is stronger. This coupling term is traditionally called the "matrix element" for the transition: this term comes from an alternative formulation of quantum mechanics in terms of matrices rather than the differential equations of the Schrodinger approach. The matrix element can be placed in the form of an integral where the interaction which causes the transition is expressed as a potential V which operates on the initial state wavefunction. The transition probability is proportional to the square of the integral of this interaction over all of the space appropriate to the problem.

This kind of integral approach using the wavefunctions is of the same general form as that used to find the "expectation value" or expected average value of any physical variable in quantum mechanics. But in the case of an expectation value for a property like the system energy, the integral has the wavefunction representing the eigenstate of the system in both places in the integral.

The transition probability is also proportional to the density of final states r_f. It is reasonably common for the final state to be composed of several states with the same energy - such states are said to be "degenerate" states. This degeneracy is sometimes expressed as a "statistical weight" which will appear as a factor in the transition probability. In many cases there will be a continuum of final states, so that this density of final states is expressed as a function of energy.