Energy to Confine Particles in a Carbon Atom

The carbon atom, in particular an atom of the isotope ¹⁴C, has been chosen to illustrate the implications of quantum mechanics for the energy required to confine a particle to a region of space. Without recourse to the details of the nature of the fundamental forces, quantum mechanics gives the insight that it takes 10's of electron volts to contain electrons in atoms, and energies on the order of MeVs to contain protons in nuclei.

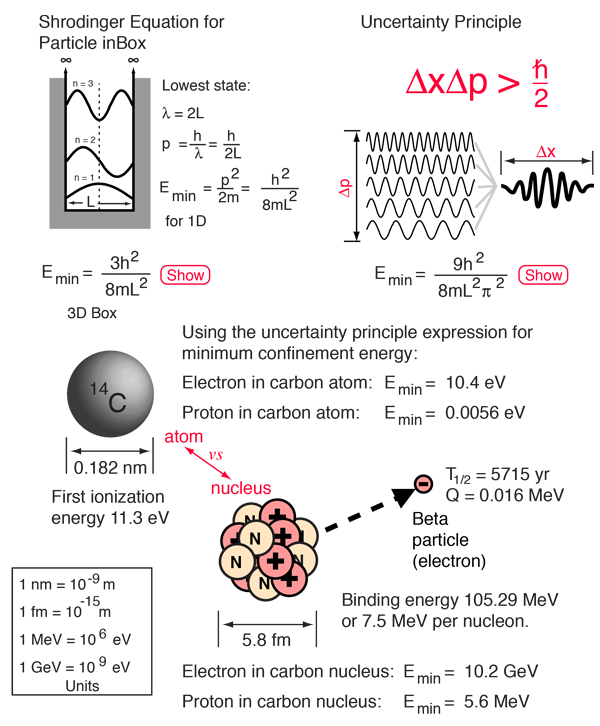
Taking the diameter of a carbon atom from the periodic table and calculating the minimum energy consistent with the uncertainty principle for a cubical volume of that dimension, we obtain a value of 10.4 eV. This compares with the observed value of 11.3 eV for the first ionization potential for the carbon atom. If we calculate the minimum confinement energy for the proton in a space the size of a carbon atom, we find that to be only 0.0056 eV. This extremely low energy can be compared with the average thermal energy of 0.04 eV at 300K! This implies that the proton, with only the energy provided by the internal energy of the normal environment can just wander in and out of an atomic space.

When we apply the minimum energy calculation to the carbon nucleus, the picture is very different. The confinement energy for keeping a proton inside the a cubical volume of dimension equal to the nuclear diameter is 5.6 MeV. This compares well in magnitude with the observed average binding energy of 7.5 MeV for nucleons in the carbon-14 nucleus. So with no tools other than the uncertainty principle, we have established the scale of energy for nuclear processes. Observed radioactive processes are in the range 0-10 MeV, and this is consistent with the uncertainty principle.

The story for the electron is more dramatic. We observe electrons being emitted from the carbon-14 nucleus (beta decay) with the relatively small energy of 0.016 MeV. Does that imply that there are electrons hanging around inside the carbon nucleus? Definitely not!! The minimum confinement energy for an electron in the nuclear volume is a preposterously high 10.2 GeV, a half-million times greater than the observed decay energy of the carbon-14 nucleus. The calculation of required confinement energy then implies that the observed electron has been created inside the nucleus as a part of the radioactive decay process rather than being simply an ejection of an electron which was already there.