Corona from Fogged Eyeglasses

When light from a point source passes through a small circular aperture, it does not produce a bright dot as an image, but rather a diffuse circular disc known as Airy's disc surrounded by much fainter concentric circular rings. This example of diffraction is of great importance because the eye and many optical instruments have circular apertures. Diffraction also occurs in the inverse situation when you have a small opaque circle. The corona sometimes seen around the moon is attributed to diffraction from small water droplets or ice crystals.

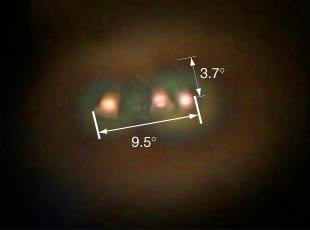
The image above seems to be the same phenomenon observed when my eyeglasses were fogged upon entering the warm house after walking the dog on a cold January morning. It is attributed to diffraction from very tiny water droplets which condensed from the fairly dry room air. This diffraction pattern might be used to estimate the size of the condensed water droplets, but the two images below exhibit different colors in the inner part of the diffraction pattern and suggest that different orders of diffraction are manifested in the color patterns.

The two images above were taken within seconds of each from areas on the fogged eyeglasses probably within a centimeter of each other. The different color patterns are taken as evidence of different sized droplets, although it is not clear what order of diffraction is being manifested.

Another phase of the fogged eyeglass experiment was facilitated by the fact that my wife had a nice pot of bean soup boiling on the kitchen stove on this cold morning. Another short walk around the cold neighborhood and then putting my glasses above the boiling pot produced a fog which did not exhibit the colored diffraction rings. Presumably the hot saturated air rising from the soup formed larger droplets which did not show the visible diffraction rings - the larger the aperture, the smaller the diffraction pattern. The scattered light in the left image above showed a symmetric decrease in intensity as you moved further from the light. It might be properly described as Mie scattering. At right above is an image taken once the fog on my glasses had time to partially evaporate. There is evidence of diffraction here in the slight modulation of the intensity close to the lights. This is taken as evidence that the evaporation process was leaving smaller droplets which were beginning to produce visible diffraction effects.

Corona from Fogged Eyeglasses: Estimation of Droplet Size

From an image of aperture diffraction formed by fog droplets on cold eyeglasses, an estimate of droplet size can be made. The colored fringes are presumed to be droplet diffraction like that sometimes seen as a corona around the moon. The glasses were positioned at 3.66 m (12 ft) and the horizontal span of the lights was 0.6 m (2 ft). The span of the lights then represents an angle of 9.5°. Scaling the image gave an angle of 3.7° out to the red fringe.

For a first estimate, assume a wavelength of about 600 nm for the red fringe and assume that the red fringe represents the first subsidiary maximum of the aperture diffraction. Using the aperture calculation with 3.7° as the first maximum gives a circular aperture diameter of about 15 microns. If that represents the diameter of the fog droplets, then that is a measure of 15 microns for the droplet size.

There are many reasons for caution about claiming that the above estimate represents a real measurement of droplet size. One of them is illustrated in the two images above. Two images taken within seconds of each other and very close together spatially show different diffraction patterns. The one on the left has a red fringe about half as far out as that one used in the estimate above, which would give droplet size 30 microns. While that may be reasonable, one cannot be sure that it is the first subsidiary maximum which is giving the color to the fringe. If the red fringe in the first estimate above were the second subsidiary maximum, then the estimated size would be 25 microns and if it were the third, the estimate would be 34 microns.

A further reason for caution about claims for particle size is that the diffraction is occuring around a droplet which is itself partially transparent. The diffraction pattern is not in fact the same as that expected from a circular opaque barrier. Experiences with estimating the diameter of human hairs by measuring the diffraction from them has led to caution about claiming to have measured size from the diffraction.

A similar phenomenon, also attributed to aperture diffraction from small particles, is the set of colored fringes one can sometimes see around a bright light source like a lightbulb. This corona effect is attributed to small particles on the surface of your eye. My personal observation of this effect is usually early in the morning. The act of blinking changes the intensity of the fringes and they disappear after a few minutes of blinking, suggesting that they are surface phenomena. One particularly prominent set of colored fringes was measured by locating the center of the prominent red ring at about 15 cm from the center of the light I was looking at. The distance to the light was 2.44 meters. The red color seemed to be of a similar hue to a helium-neon laser, so a wavelength of 630 nm was estimated. Assuming that the prominent red ring was the first subsidiary maximum of the aperture diffraction gives a calculated aperture size of 16 micrometers. This estimate is certainly subject to the uncertainties described above in regard to the fog droplets.

Meyer-Arendt in Section 3.5 of Introductory Classical and Modern Optics, 3rd Ed. reports his personal measurement and arrives at a particle diameter of 12.4 micrometers. He suggests that it might be the epithelium cells of the cornea that cause the effect.