Image from Principal Planes

The thin lens equation can be used with thick lenses if the principal planes are found. The equivalent focal length of the thick lens can be found from Gullstrand's equation, and the front and back vertex focal lengths obtained. Once that is done, the principal planes can be located by subtracting the vertex focal lengths from the equivalent focal length. The equivalent focal length and the distance from first principal distance H₁ to the object can be used to calculate the image distance from the second principal plane H₂. From that distance, the image distance from the back vertex can be deduced.

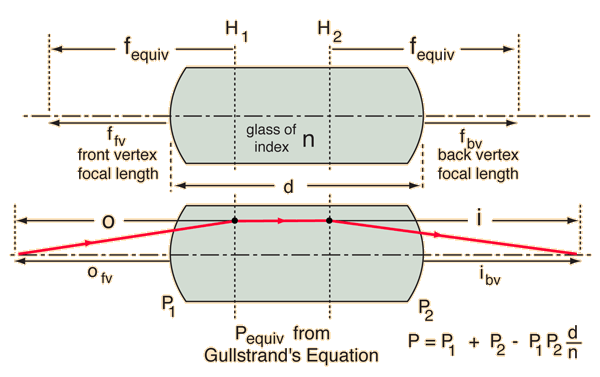
Note that the calculation below does not take into account the change in lens thickness with the angle of the incoming ray. It is typical to do the calculation only for the paraxial rays where the departure from full thickness is negligible. The segments in boxes below are the limiting cases where the lens thickness is taken as zero (thin lens approximation).

For glass with index of refraction n_lens =

the front surface power for lens radius R₁ = m is P₁ = m^-1

and the back surface power for lens radius R₂ = m is P₂ = m^-1

(Note that for a double convex lens, the front surface radius R₁ is positive and the back surface radius R₂ is negative according to the Cartesian sign convention.)

A thin lens would have approximate power P_{thin lens} = P₁ + P₂ = m^-1.

The thin lens focal length would be f = m = cm.

A thick lens of thickness d = cm = m would have effective power given by Gullstrand's equation:

P_{thick lens} = m^-1

The equivalent focal length for the thick lens would be f_equiv = m = cm.

Sometimes for thick lenses it is more useful to state the back vertex power and focal length. For this lens:

P_{front vertex} = m^-1.

Front vertex focal length = f_{front vertex} = m = cm.

P_{back vertex} = m^-1.

Back vertex focal length = f_{back vertex} = m = cm.

For the thin lens, we can calculate the image distance from the simpler form of the lens equation. For object distance

o = m, the image distance is

i = m.

Remember that an object in front of the lens gives a negative object distance. Also remember that an object inside the focal length does not form a real image. A negative image distance impies a virtual image.

For the thick lens, we will specify the distance of the object from the front surface of the lens:

o_fv = m.

To use the lens equation for the thick lens, we must find the object distance to the first principal plane H₁, so we must calculate the distance from the front vertex to that plane. That distance is the difference between the equivalent focal length and the front vertex focal length, f_equiv - f_fv.

The distance from the front vertex to H₁ = m so o_{thick lens} = m.

The lens equation, using P_{thick lens}, then gives the image distance

i_{thick lens} = m.

This locates the image for us, but if we want the image distance from the back vertex, we have to calculate the distance from H₂ to that vertex. That distance is the difference between the equivalent focal length and the back vertex focal length, f_equiv - f_bv.

H₂ to back vertex = m.

The image distance from the back vertex is then

i_bv = m.

Note that the use of the lens equation in this setting leaves out the separation between the principal planes.

H₂ - H₁ = m.

The total distance from the object to the image for the thick lens is

o_thick + (H₂ - H₁) + i_thick = m.

For the thin lens, this distance would be just o+i = m. In many practical cases this distance is very close to just the thick lens total distance minus the thickness of the lens.

Compare to same calculation by:

Vergence tracing

Principal planes, two thin lenses

Vergence Example

Matrix definitions

Index

Lens concepts

Thick lens concepts

Reference
Meyer-Arendt

HyperPhysics***** Light and Vision

R Nave

Go Back