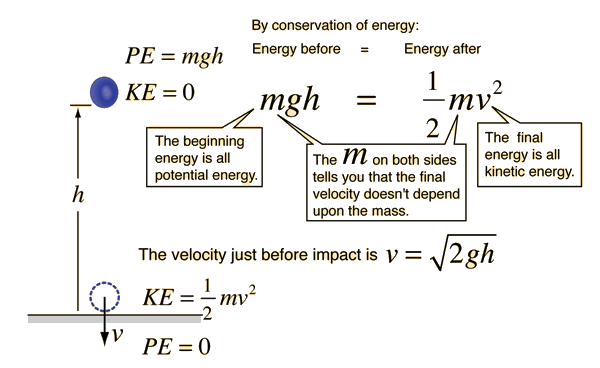Energy as a tool for mechanics problem solving
The application of the conservation of energy principle provides a powerful tool for problem solving. Newton's laws are used for the solution of many standard problems, but often there are methods using energy which are more straightforward. For example, the solution for the impact velocity of a falling object is much easier by energy methods. The basic reason for the advantage of the energy approach is that just the beginning and ending energies need be considered; intermediate processes do not need to be examined in detail since conservation of energy gaurantees that the final energy of the system is the same as the initial energy.
The work-energy principle is also a useful approach to the use of conservation of energy in mechanics problem solving. It is particularly useful in cases where an object is brought to rest as in a car crash or the normal stopping of an automobile.
|