Power Transfer to a Resistive Load
As a general rule, the maximum power transfer from an active device like a power supply or battery to an external device occurs when the impedance of the external device matches that of the source. That optimum power is 50% of the total power when the impedance of the active device is matched to that of the load. Improper impedance matching can lead to excessive power use and possible component damage. This situation will be modeled here for strictly resistive impedances.
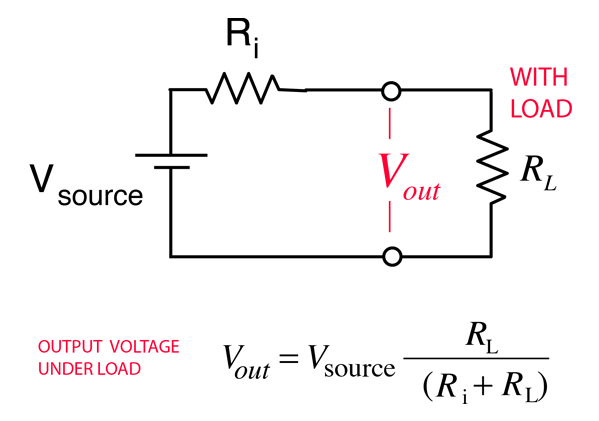
This is an important practical situation in DC circuits, enabling you to model the output of batteries with internal resistance and other situations where the power supply has internal resistance. Note that the power output from the voltage source, which is assumed to be ideal, is maximum when the load resistor RL is equal to the internal resistance Ri, delivering 50% of the source power to the load. You can get a higher percentage of the power to the load by increasing the load resistor, and that is the desirable situation with a battery with low internal resistance. Any power used up in the internal resistor is lost to heat. But the absolute power to the load will be diminished.
Note: To avoid dealing with so many short circuits, divider resistors with value zero will default to 1 when the voltage is changed. They can be changed back to a zero value if you wish to explore the effects of short circuits. Ohms are indicated as the resistance unit, but kilohms are more common (giving powers in milliwatts) and of course the numerical calculation is the same.
| DC circuit examples | AC voltage divider |
DC Circuits
| HyperPhysics***** Electricity and Magnetism | R Nave |